Remarkable identity

In mathematics, a necessary step, remarkable identities.

Why learn remarkable identities in mathematics.
What can this bring us?

Normally, the goal of retaining this type of equality is to speed up calculations, and ultimately to find a possible solution to an equation.

The fact that the remarkable identity already presents a ready-made calculation can actually make it possible to simplify the equation and ultimately to deduce a result more quickly.

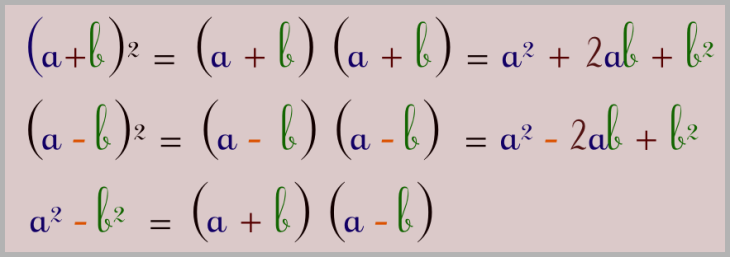
The main identities are:

(a+b)² = a² + 2ab + b²
(a-b)² = a² – 2ab + b²

the calculation is done as follows:

(a+b)² = (a+b) (a+b) = a² + 2ab + b²
(a-b)² = (a-b) (a-b) = a² – 2ab + b²
a² – b² = (a+b) (a-b)